Introduction to True Statements
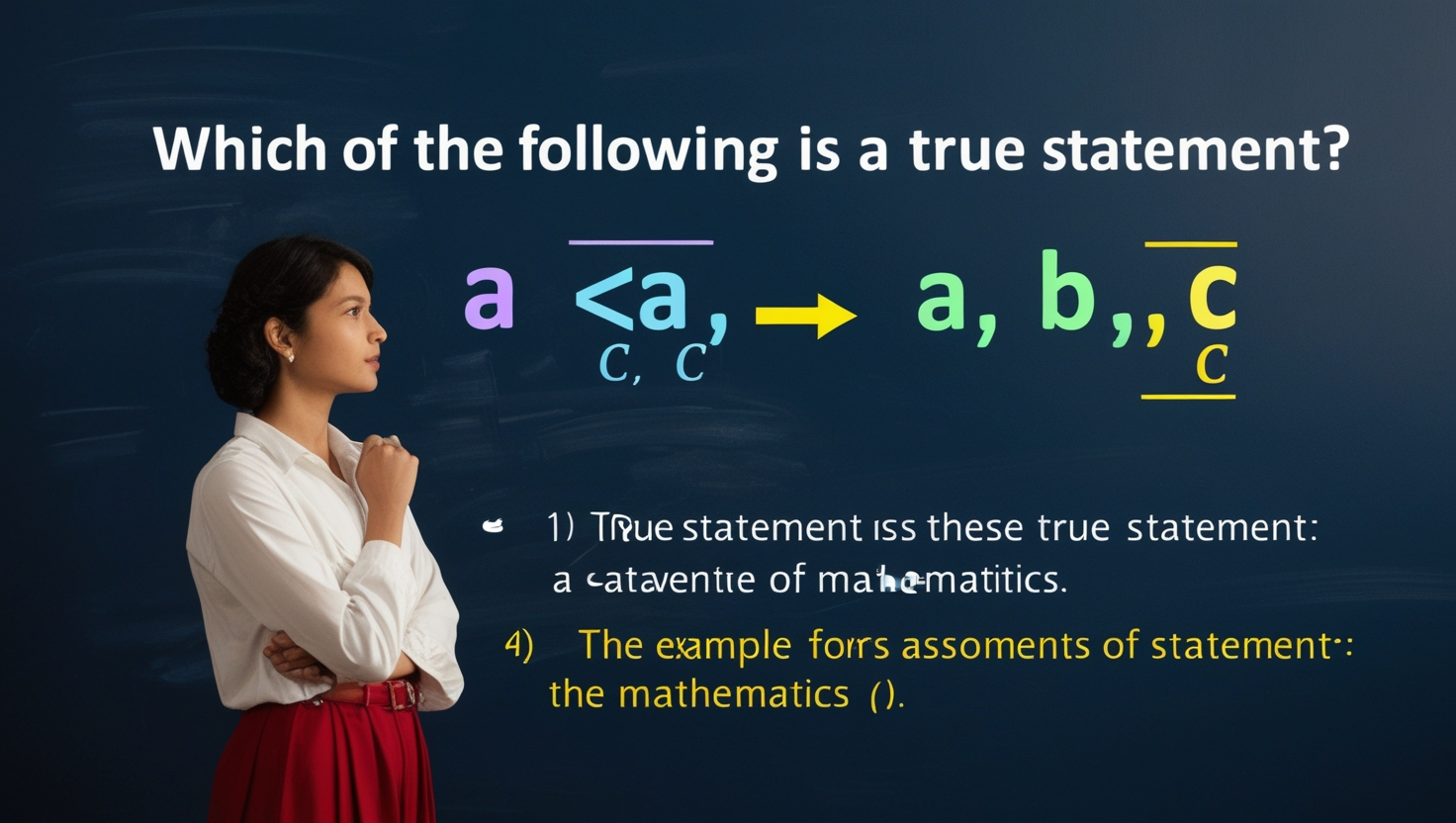
In the realm of mathematics, the concept of truth is foundational. It forms the basis upon which logical arguments are constructed and validated. When we encounter the phrase “Which of the following is a true statement,” we are tasked with discerning the correctness of various propositions. This article will delve into the nature of factual statements, mainly focusing on the statement “{a} ⊆ {a, b, c}” as a prime example. By exploring the properties and implications of factual statements, we aim to provide a comprehensive understanding of their role in mathematical reasoning.
What Defines a True Statement?
A true statement accurately reflects reality or conforms to the rules of logic and mathematics. In simpler terms, it is a statement that is correct in all instances within its defined context. For example, the assertion “the number three is always equal to three” is accurate because it is universally valid and consistent with the definition of equality in arithmetic. When inquired, “Which of coming up next is a genuine assertion?
The Importance of True Statements
Factual statements are crucial in mathematics because they are the building blocks for more complex theorems and proofs. With a foundation of factual statements, it is possible to construct reliable and valid mathematical arguments. For instance, the statement “{a} ⊆ {a, b, c}” is true because the set {a} is indeed a subset of {a, b, c}. This truth is vital for understanding set theory and its applications in various mathematical disciplines.
Analyzing the Assertion “{a} ⊆ {a, b, c}”
To better understand why “{a} ⊆ {a, b, c}” is a true statement, we need to explore the concept of subsets. A set An is viewed as a subset of a set B if each component of An is likewise a component of B. For this situation, the set {a} contains just a single component, ‘a’, likewise present in the set {a, b, c}. Therefore, {a} is indeed a subset of {a, b, c}, making the statement “{a} ⊆ {a, b, c}” unequivocally true.
The Role of Context in Determining Truth
The truth of a statement in mathematics often depends on the context and the definitions provided. For example, in the context of set theory, the statement “{a} ⊆ {a, b, c}” is true based on the definition of subsets. However, if we were to change the context or alter the definitions, the statement’s truth value could change. This highlights the importance of clearly defining terms and conditions when evaluating the truth of mathematical statements.
Factual Statements vs. False Statements
In contrast to factual statements, false statements do not hold under the given conditions. A report is considered false if it can be proven incorrect in at least one instance. For example, the statement “{d} ⊆ {a, b, c}” is false because the element ‘d’ is not present in the set {a, b, c}. When determining “which of the following is a true statement,” it is essential to carefully analyze each proposition and verify its correctness based on the given definitions and conditions.
The Concept of Open Statements
Apart from authentic and false statements, mathematics also deals with open statements. An open assertion is one whose reality esteem relies upon an obscure or variable component. For example, the statement “x is more imperative than 5” is open because its world depends upon the value of x. Assuming x is 6, the explanation is valid; on the off chance that x is 4, the articulation is bogus. Open statements become true or false only when the unknown element is specified or defined.
Practical Examples of True Statements
To further illustrate the concept of factual statements, consider the following examples:
- “2 + 2 = 4” – This arithmetic statement is accurate because it correctly represents the sum of two and two.
- “A triangle has three sides” – This geometric statement is true based on the definition of a triangle.
- “{1, 2} ⊆ {1, 2, 3}” – This set theory statement is true because both elements of the first set are present in the second set.
When evaluating “which of the following is a true statement,” these examples clearly illustrate universally valid propositions.
The Importance of Logical Consistency
Factual statements contribute to the logical consistency of mathematical systems. Mathematicians can build reliable frameworks for solving complex problems by ensuring that each statement is true. The statement “{a} ⊆ {a, b, c}” exemplifies this principle by demonstrating the consistent application of the subset definition. Maintaining logical consistency is essential for the integrity of mathematical reasoning and the validity of conclusions drawn from it.
Identifying True Statements in Practice
Identifying factual statements is a critical skill in practical applications. Whether solving equations, proving theorems, or analyzing data, the ability to discern true from false statements underpins successful mathematical practice. When presented with multiple propositions, asking, “Which of the following is a true statement?” helps focus on the correct and relevant information, facilitating accurate problem-solving and decision-making.
Conclusion: The Power of True Statements
Factual statements are the cornerstone of mathematical logic and reasoning. They provide the foundation for constructing valid arguments, proving theorems, and solving problems. The statement “{a} ⊆ {a, b, c}” is a prime example of a true statement, illustrating the concept of subsets in set theory. By understanding and applying factual statements, mathematicians and students alike can ensure the accuracy and reliability of their work. When faced with the question, “Which of the following is a true statement,” the principles discussed in this article offer a clear path to identifying and validating true propositions, reinforcing the integrity of the mathematical inquiry.